nLab line with two origins
Context
Topology
topology (point-set topology, point-free topology)
see also differential topology, algebraic topology, functional analysis and topological homotopy theory
Basic concepts
-
fiber space, space attachment
Extra stuff, structure, properties
-
Kolmogorov space, Hausdorff space, regular space, normal space
-
sequentially compact, countably compact, locally compact, sigma-compact, paracompact, countably paracompact, strongly compact
Examples
Basic statements
-
closed subspaces of compact Hausdorff spaces are equivalently compact subspaces
-
open subspaces of compact Hausdorff spaces are locally compact
-
compact spaces equivalently have converging subnet of every net
-
continuous metric space valued function on compact metric space is uniformly continuous
-
paracompact Hausdorff spaces equivalently admit subordinate partitions of unity
-
injective proper maps to locally compact spaces are equivalently the closed embeddings
-
locally compact and second-countable spaces are sigma-compact
Theorems
Analysis Theorems
Contents
Idea
What is called the line with two origins is the topological space which results by “gluing” a copy of the real line identically to itself, except at the origin. This is a basic example of a non-Hausdorff topological space (even a non-Hausdorff smooth manifold) and of the fact that quotient topological spaces of Hausdorff topological spaces need not be Hausdorff themselves.
Definition
Consider the disjoint union of two copies of the real line regarded with its metric topology. Moreover, consider the equivalence relation on the underlying set which identifies every point in the th copy of () with the corresponding point in the other, the th copy, except when :
The quotient topological space
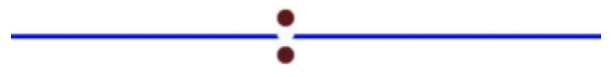
by this equivalence relation is called the line with two origins.
Properties
The line with two origins is a non-Hausdorff topological space:
Because by definition of the quotient space topology, the open neighbourhoods of are precisely those that contain subsets of the form
But this means that the “two origins” and may not be separated by neighbourhoods, since the intersection of with is always non-empty:
of the line with two origins is the real line itself:
However, the line with two origins is T1 and sober.
It is also a topological manifold, even a smooth manifold, except that one often requires such to be Hausdorff to rule out examples such as this. In a similar vein, it is locally compact (and a compact version can be made by starting with instead of ), paracompact, and so forth.
Related concepts
References
- Wikipedia, Line with two origins
Last revised on September 19, 2021 at 04:58:40. See the history of this page for a list of all contributions to it.