nLab infinitesimal braid relation

Definition

Definition

(infinitesimal braid Lie algebra)

For $n \in \mathbb{N}$ natural numbers, let $F(\{t_{i j}\}_{i\neq j \in \{1,\cdots, n\}})$ be the free Lie algebra (over a given ground field) on generators $t_{i j}$ in degree with two indices ranging from 1 to $n$ and distinct.

The infinitesimal braid relations in dimension $D = 2$ are the following relations on the underlying vector space of the free Lie algebra on generators $\{t_{i j}\}_{i\neq j \in \{1,\cdots, n\}}$

(1)$$\left. \begin{array}{lcll} R0: \;\; & t_{i j} - t_{j i} & = 0 \\ R1: \;\; & [t_{i j}, t_{k l}] & = 0 \\ R2: \;\; & [t_{i j}, t_{i k} + t_{k j}] & = 0 & \mathrlap{\,} \end{array} \right\} \text{for pairwise distinct indices} \;\;$$

This defines a quotient Lie algebra often denoted

$$\mathcal{L}_n \;\coloneqq\; F(\{t_{i j}\}_{i\neq j \in \{1,\cdots, n\}}) /(R0, R1, R2) \,.$$

More generally, there is a version of this in any dimension $D \in \mathbb{N}$ with some extra signs thrown in.

This is originally due to Kohno 87 (1.1.4), it appears also in Kohno 88, Bar-Natan 96, Fact 3, Fadell-Husseini 01, Cohen-Gitler 01, Section 3.

Authors have used different equivalent versions of this presentation, see Cohen-Gitler 02, p. 2 for a clear statement.

Properties

Relation to horizontal chord diagrams

If $F(\{t_{i j}\}_{i\neq j \in \{1,\cdots, n\}})/R0$ is identified with the commutator Lie algebra of horizontal chord diagrams on $n$ strands, generated by

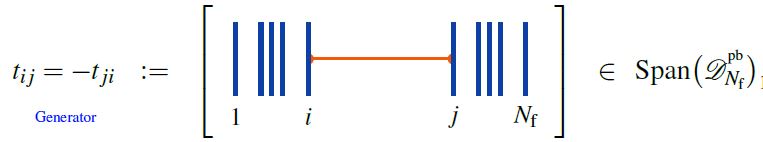
and forming an associative algebra under concatenation of diagrams along strands, as in

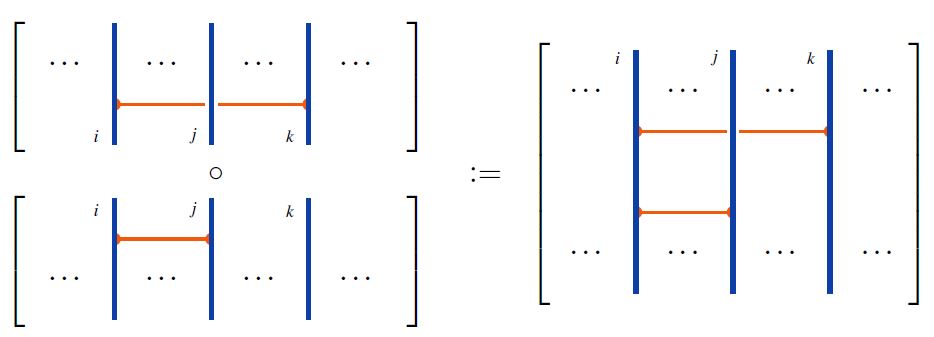
then the remaining infinitesimal braid relations (1) are equivalently the following:

R1 is the the 2T relation:

R2 is the 4T relation:

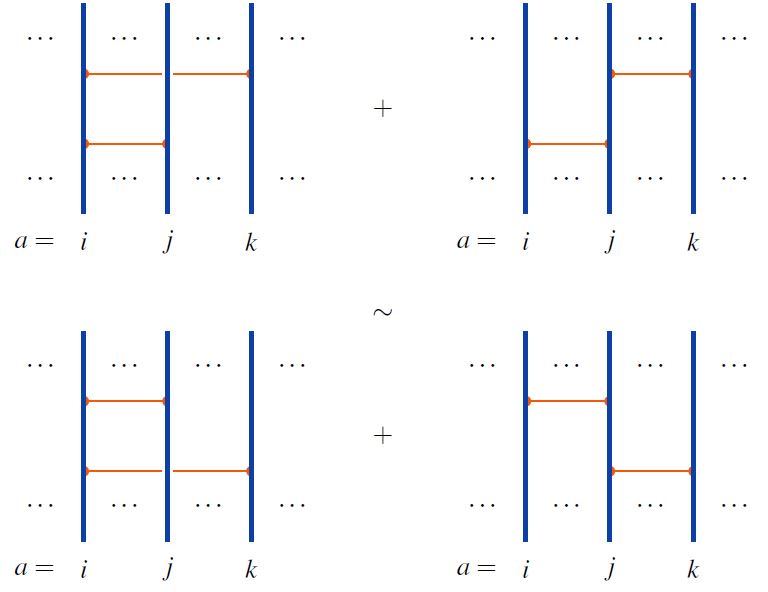
graphics from Sati-Schreiber 19c

Hence:

Proposition

(universal enveloping algebra of infinitesimal braid Lie algebra is horizontal chord diagrams modulo 2T&4T)

The associative algebra

$$\Big( \mathcal{A}_n^{pb} \;\coloneqq\; Span \big( \mathcal{D}_n^{pb} \big)/(2T, 4T) , \circ \Big)$$

of horizontal chord diagrams on $n$ strands with product given by concatenation of strands (this Def.), modulo the 2T relations and 4T relations (this Def.) is isomorphic to the universal enveloping algebra of the infinitesimal braid Lie algebra (Def. ):

$$\big(\mathcal{A}_n^{pb}, \circ\big) \;\simeq\; \mathcal{U}(\mathcal{L}_n(D)) \,.$$

Related concepts

• braid group

• Yang-Baxter equation

• weight systems are cohomology of loop space of configuration space

chord diagrams	weight systems
linear chord diagrams, round chord diagrams Jacobi diagrams, Sullivan chord diagrams	Lie algebra weight systems, stringy weight system, Rozansky-Witten weight systems

knots	braids
chord diagram, Jacobi diagram	horizontal chord diagram
1T&4T relation	2T&4T relation/ infinitesimal braid relations
weight system	horizontal weight system
Vassiliev knot invariant	Vassiliev braid invariant
weight systems are associated graded of Vassiliev invariants	horizontal weight systems are cohomology of loop space of configuration space

References

• Toshitake Kohno, (1.1.4) in: Monodromy representations of braid groups and Yang-Baxter equations, Annales de l’Institut Fourier, Volume 37 (1987) no. 4, p. 139-160 (doi:10.5802/aif.1114)

• Toshitake Kohno, Linear representations of braid groups and classical Yang-Baxter equations, in: Joan S. Birman, Anatoly Libgober (eds.) Braids Cont. Math. 78 (1988), 339-363 (doi:10.1090/conm/078)

• Dror Bar-Natan, Fact 3 in: Vassiliev and Quantum Invariants of Braids, Geom. Topol. Monogr. 4 (2002) 143-160 (arxiv:q-alg/9607001)

• Edward Fadell, Sufian Husseini, Theorem 2.2 in: Geometry and topology of configuration spaces, Springer Monographs in Mathematics (2001), MR2002k:55038, xvi+313

• Fred Cohen, Samuel Gitler, Section 3 in: Loop spaces of configuration spaces, braid-like groups, and knots, In: Jaume Aguadé, Carles Broto, Carles Casacuberta (eds.) Cohomological Methods in Homotopy Theory. Progress in Mathematics, vol 196. Birkhäuser, Basel 2001 (doi:10.1007/978-3-0348-8312-2_7)

• Fred Cohen, Samuel Gitler, p. 2 of: On loop spaces of configuration spaces, Trans. Amer. Math. Soc. 354 (2002), no. 5, 1705–1748, (jstor:2693715, MR2002m:55020)